Proofs Without Words

I guess there's nothing much to explain on this one.

One can easily see that the length and breadth of the above rectangle are $\mathcal{F_{n+1}}$and $\mathcal{F_{n}}$. Area of the rectangle = $\sum$ Area of the squares or, $$\mathcal{F_{n}F_{n+1}=F_{0}^{2}+F_{1}^{2}+\cdots+F_{n}^{2}}$$

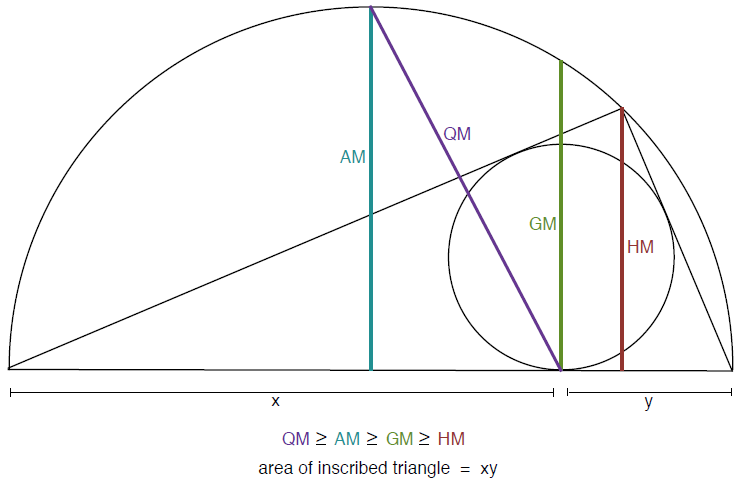
Take any 2 circles among the given blue circles. Draw 2 lines inclined at $60 ^{\circ}$ to each other . For every such pair of points we get a corresponding yellow point. If the total number of blue circles is $n$,the number of ways to select 2 circles = $\binom{n}{2}$. Count the total number of yellow circles. Now I guess it is easy to see that: $$1+2+\cdots + (n-1) = \binom{n}{2}$$

Without loss of generality we may assume $a\leq b\leq c$. It is quite obvious that $$a+c\geq b\qquad a+b>c$$ Rotate $\mathcal{BC}$ about $\mathcal{B}$ and $\mathcal{AC}$ about $\mathcal{A}$. Now as we can see in the above diagram $$a+b>c$$ NOTE: $a+b=c$ would imply that the triangle is degenerate.

        Let the side of each of the 4 innermost squares be 1. Total area of these squares=$4\times 1\times 1=4\cdot (1)^{3}$ Total area of the squares coloured purple = $8\times 2\times 2= 4\cdot (2)^{3}$ Total area of the squares coloured pink = $12\times 3\times 3= 4\cdot (3)^{3}$ Total area of the outermost squares = $16\times 4\times 4= 4\cdot (4)^{3}$   Total area of all the squares = $$4(1^{3}+2^{3}+3^{3}+4^{3})=4\sum n^{3}$$   The total area is also = {(Side of the outermost square)$\times$(Number of outermost squares accross the length)}$^{2}$   = $(5\times 4)^{2}$ = ${n(n+1)}^{2}$ Therefore, $$\sum n^{3}=\left[\frac{n(n+1)}{2}\right]^{2}=(\sum n)^{2}$$

Proof of $$1+3+5+\cdots = n^{2}$$ Count the number of balls in the L shaped regions.Notice that they are odd numbers. Furthermore,count the number of balls considering the length or the breadth(they will turn out to be the same). Sum of all balls = (number of balls on the length)$^{2}$.