Sum of cubes

 

 

 

 

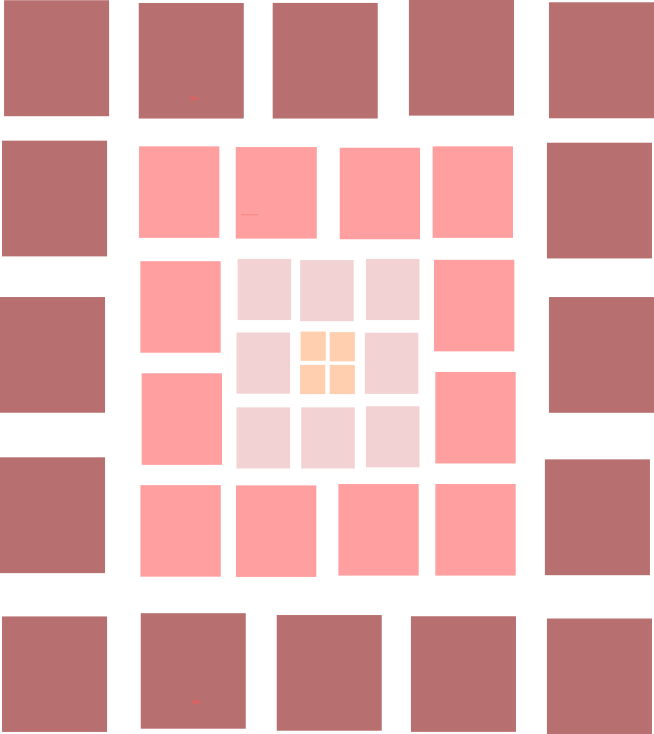
Let the side of each of the 4 innermost squares be 1.

Total area of these squares=$4\times 1\times 1=4\cdot (1)^{3}$

Total area of the squares coloured purple = $8\times 2\times 2= 4\cdot (2)^{3}$

Total area of the squares coloured pink = $12\times 3\times 3= 4\cdot (3)^{3}$

Total area of the outermost squares = $16\times 4\times 4= 4\cdot (4)^{3}$

 

Total area of all the squares = $$4(1^{3}+2^{3}+3^{3}+4^{3})=4\sum n^{3}$$

 

The total area is also =

{(Side of the outermost square)$\times$(Number of outermost squares accross the length)}$^{2}$





= $(5\times 4)^{2}$ = ${n(n+1)}^{2}$

Therefore,

$$\sum n^{3}=\left[\frac{n(n+1)}{2}\right]^{2}=(\sum n)^{2}$$